Artur Sergyeyev: Difference between revisions
No edit summary |
No edit summary |
||
| (16 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
The search for partial differential systems in four independent variables ('''(3+1)D''' | |||
for short) that are ''integrable'' in the sense of soliton theory a.k.a. ''exactly solvable'' is a longstanding open | |||
problem of mathematical physics as according to general relativity our spacetime is | |||
four-dimensional, and thus the (3+1)D case is particularly relevant for applications. | |||
This problem is addressed in a positive fashion in my recent article | |||
A. Sergyeyev, [https://arxiv.org/abs/1401.2122 ''New integrable (3+1)-dimensional systems and contact geometry''], | |||
Lett. Math. Phys. '''108''' (2018), no. 2, 359-376 ([https://arxiv.org/abs/1401.2122 arXiv:1401.2122]) | |||
where it is shown that integrable (3+1)D systems are significantly less exceptional than | |||
it appeared before: in addition to a handful of well-known important yet isolated examples | |||
like the (anti)self-dual Yang--Mills equations there is a large new class of integrable | |||
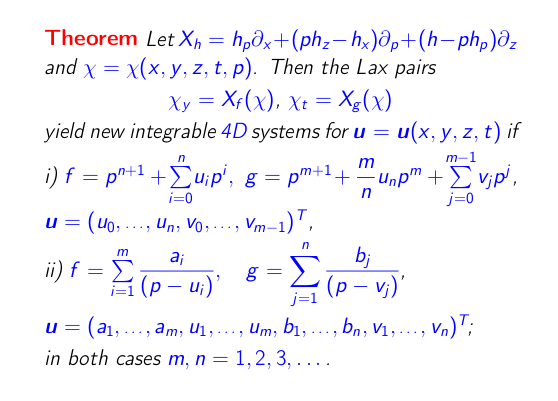
(3+1)D systems with Lax pairs of a novel kind related to contact geometry: | |||
[[File:thm.png]] | |||
Please see the article for the explicit form of two infinite families of integrable (3+1)D systems from the above picture. | |||
For example, system (40) from the article in question | |||
is a new (and the only known to date) integrable generalization from three to four | |||
independent variables for the Khokhlov--Zabolotskaya equation, also known as | |||
the dispersionless Kadomtsev--Petviashvili equation or the Lin--Reissner--Tsien | |||
equation and having many applications in nonlinear acoustics and fluid dynamics. | |||
Moreover, within the above new class of integrable (3+1)D systems we found what is, | |||
to the best of our knowledge, a first example of an integrable (3+1)D system with a | |||
nonisospectral Lax pair that involves algebraic, rather than merely rational, dependence | |||
on the spectral parameter. This result shows inter alia that the class of integrable (3+1)D | |||
dispersionless systems with nonisospectral Lax pairs is significantly more diverse than | |||
it appeared before. For further details please see the paper | |||
A. Sergyeyev, [https://authors.elsevier.com/a/1YWkT3BGwf3whj ''Integrable (3+1)-dimensional system with an algebraic Lax pair''], | |||
Appl. Math. Lett. 92 (2019), 196-200 ([https://arxiv.org/abs/1812.02263 arXiv:1812.02263]) | |||
You may wish to look at these [https://figshare.com/articles/Multidimensional_Integrability_via_Geometry/7531529 slides] for additional background and motivation before proceeding to the articles themselves. | |||
[https://sites.google.com/site/artursergyeyev/ My main page] | |||
[[Category: People|Sergyeyev]] | [[Category: People|Sergyeyev]] | ||
Latest revision as of 06:47, 24 February 2019
The search for partial differential systems in four independent variables ((3+1)D for short) that are integrable in the sense of soliton theory a.k.a. exactly solvable is a longstanding open problem of mathematical physics as according to general relativity our spacetime is four-dimensional, and thus the (3+1)D case is particularly relevant for applications.
This problem is addressed in a positive fashion in my recent article
A. Sergyeyev, New integrable (3+1)-dimensional systems and contact geometry, Lett. Math. Phys. 108 (2018), no. 2, 359-376 (arXiv:1401.2122)
where it is shown that integrable (3+1)D systems are significantly less exceptional than it appeared before: in addition to a handful of well-known important yet isolated examples like the (anti)self-dual Yang--Mills equations there is a large new class of integrable (3+1)D systems with Lax pairs of a novel kind related to contact geometry:
Please see the article for the explicit form of two infinite families of integrable (3+1)D systems from the above picture. For example, system (40) from the article in question is a new (and the only known to date) integrable generalization from three to four independent variables for the Khokhlov--Zabolotskaya equation, also known as the dispersionless Kadomtsev--Petviashvili equation or the Lin--Reissner--Tsien equation and having many applications in nonlinear acoustics and fluid dynamics.
Moreover, within the above new class of integrable (3+1)D systems we found what is, to the best of our knowledge, a first example of an integrable (3+1)D system with a nonisospectral Lax pair that involves algebraic, rather than merely rational, dependence on the spectral parameter. This result shows inter alia that the class of integrable (3+1)D dispersionless systems with nonisospectral Lax pairs is significantly more diverse than it appeared before. For further details please see the paper
A. Sergyeyev, Integrable (3+1)-dimensional system with an algebraic Lax pair, Appl. Math. Lett. 92 (2019), 196-200 (arXiv:1812.02263)
You may wish to look at these slides for additional background and motivation before proceeding to the articles themselves.